Focusing on the considerations for the contractor when encountering triplen harmonics within a three-phase circuit and the effects on current in the neutral conductor.
What are triplen harmonics and why do they affect the conductor size?
When using single-phase and/or three-phase non-linear loads such as electronic lighting ballasts/drivers and computer switch mode power supplies, typically in larger industrial/commercial type installations, the effects of harmonics within the three-phase distribution system can become more problematic, especially with the presence of ‘triplen’ harmonic currents.
As the name suggests, triplen harmonics represent multiples of the 3rd harmonic i.e., the 3rd, 6th, 9th etc. although, due to their greater impact within a three-phase installation only the odd multiples of the 3rd harmonic are normally considered – for example, the 3rd, 9th, 15th etc. This is because even harmonics (2nd, 4th, 6th etc.) are rare in AC circuits as they generally cancel out, as discussed in the article published in Issue 229 of Connections.
The example shown in Fig 1(a) highlights the 3rd harmonic current superimposed on the three-phase waveform which is in phase with all three phases. Where the harmonic crosses the zero point on all phases this is typically referred to as ‘zero sequence’.
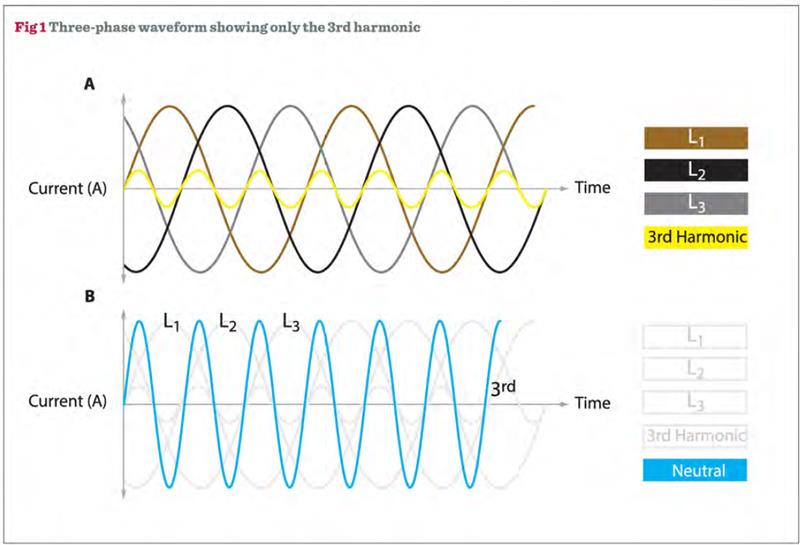
For simplicity, Fig 1(a) only shows the 3rd harmonic in phase with all three phases. However, in reality the same effect holds true for the 9th, 15th, 21st and, similarly, for all odd multiples of the 3rd harmonic. These particular harmonics are called ‘triplen’ and are the focus of Section 5.5 in Appendix 4 of BS 7671.
Considerations for the designer/installer
The main concern for installation designers/installers is that with triplen harmonics being ‘zero sequence’ the magnitude of these harmonic currents are summative in the neutral conductor, as shown in Fig 1(b). In addition, the frequency of the neutral current will also increase to roughly three times that of the supply fundamental (150 Hz), predominately through the 3rd harmonic, although the accumulation of further triplen harmonics will have significant impact.
Triplen harmonics can not only lead to distortion of the voltage sinewave but, most importantly, result in the presence of large counterproductive current in the neutral which may lead to overload unless the neutral conductor is sufficiently sized.
Typical scenario
To help provide a better understanding of the effects of triplen harmonics on circuit currents, consider the installation example shown in Fig 2. This includes a three-phase distribution board supplying three single-phase circuits, each circuit having a fundamental current of 10 A and a 3rd harmonic current of 3 A.
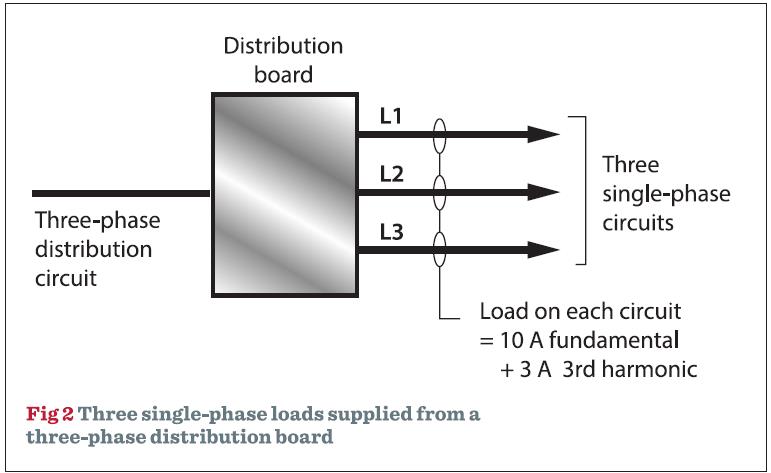
As Fig 2 indicates, it is necessary to determine the resultant current in the line and neutral conductors for each single-phase circuit before we can establish the resultant current in the line and neutral conductors of the three-phase distribution circuit.
It can be shown that for any conductor carrying harmonic current, the resultant circuit current (Irms) is given by:
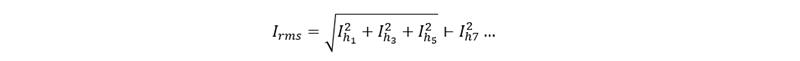
Where:
Ih1 is the rms (root mean square) value of current at the first harmonic frequency (fundamental current),
Ih1 is the third value harmonic current, etc.
In our example, the resultant current in the line and neutral conductors of each single-phase circuit is given as:
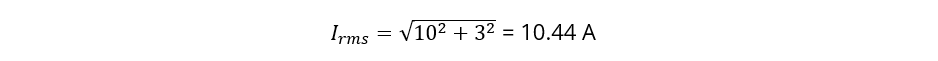
As Fig 3 indicates, this value also represents current flow in each of the line conductors supplying the distribution circuit although the resultant current in the neutral conductor of the distribution circuit still needs to be determined.
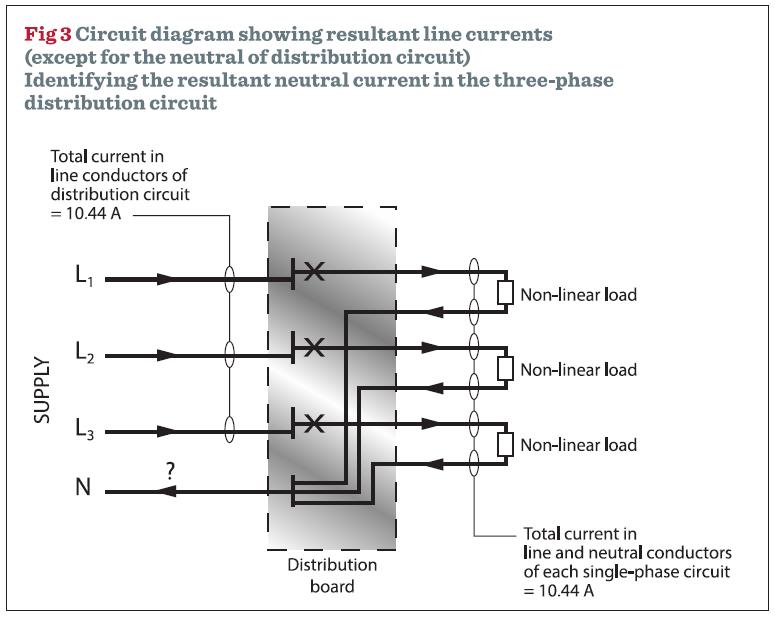
In order to establish the resultant current in the neutral conductor of the distribution circuit we must refer to the previous waveforms and consider both the fundamental and 3rd harmonic currents in all live conductors of the distribution circuit (see Fig 1(a)).
We should instinctively remember that the neutral conductor provides the return path for all three line currents, which subsequently combine in the neutral conductor. In a balanced three-phase load, the fundamental currents in the three line conductors are equal in magnitude but are displaced by 120°. As expected, these currents cancel each other out, and consequently no fundamental current flows in the neutral conductor.
However, the situation is different in our example, which includes the presence of the 3rd harmonic currents in the three line conductors. These currents are in phase with each other and equal in magnitude. Consequently, like all triplen harmonics, their magnitudes add together directly in the neutral, as shown in Fig 1B.
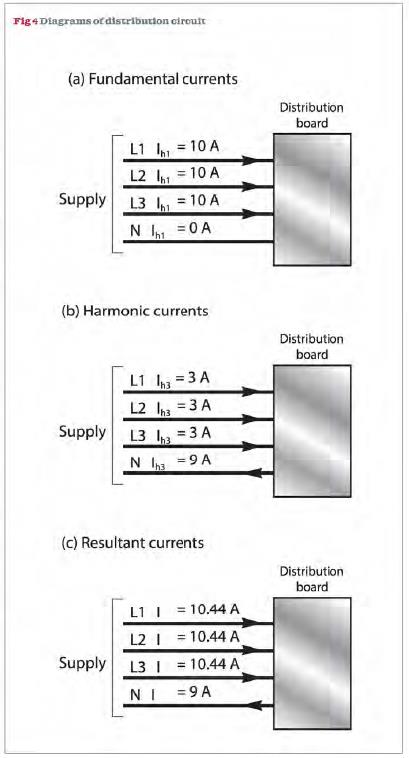
It can be said, therefore, that the resultant current in the neutral conductor of the distribution circuit is 9 A, which consists of 0 A of fundamental current for the balanced load and the contribution of the 3rd harmonic current of 3 A from each of the three line conductors. This is represented in the diagrams Fig 4(a), (b) and (c), which also show the line conductor currents and confirm that the resultant is 10.44 A, as calculated earlier.
Implications on conductor size in the presence of triplen harmonic currents
In the absence of triplen harmonics, and in a balanced system, no current would flow in the neutral conductor. However, where there is an imbalance in line conductor current, the temperature of the neutral conductor increases due to the additional current flow, although this would be offset by a reduction in the heat generated in one or more of the line conductors (523.6.2).
In comparison, the triplen harmonics present in the line conductor currents of a three-phase, four-wire circuit will not cancel in the neutral but will add together, as shown in Fig 3. The neutral conductor would therefore carry the current without a corresponding reduction in current flow in the line conductors, meaning an increase in temperature of the neutral conductor. This fundamentally reduces the current-carrying capacity of the neutral conductor and possibly that of the line conductors due to their close proximity to the neutral. This would essentially mean an increase in the minimum conductor size required for the circuit.
BS 7671 identifies the requirements for triplen harmonic current and recognises the effects on current-carrying capacity and impact on the minimum required conductor size in a three-phase, four-wire circuit, including:
- the neutral conductor must be taken into account when ascertaining the current-carrying capacity of the circuit (regulation 523.6.3 and Section 5.5 of Appendix 4 of BS 7671)
- where the total harmonic distortion due to triplen harmonics is greater than 15% of the fundamental line current:
- the neutral conductor is to be considered as a loaded conductor (523.6.1)
- the neutral conductor must not be smaller than the line conductors, as is permitted in some circumstances by regulation 524.2.3 (523.6.3).
- where the total harmonic distortion due to triplen harmonics is greater than 33% of the fundamental line conductor current, an increase may be required in the cross-sectional area (csa) of the neutral conductor (524.2.2), in which case either the neutral conductor would have to have a csa greater than that of the line conductors, or the csa of all the circuit conductors would have to be chosen taking account of the current in the neutral conductor
- the heating effect of harmonic currents on the line conductors should also be taken into account (section 5.6 of Appendix 4 of BS 7671); this includes not only triplen harmonics but other additional harmonics.
Implications on overcurrent protection
Overcurrent detection in accordance with regulation 431.2.3 must be provided for the neutral conductor where the harmonic content of the line conductor currents is such that the current in the neutral conductor might exceed the current-carrying capacity of that conductor. Therefore, harmonic currents should be taken into account when selecting an overload protective device (533.2.2).
Implications on voltage drop
Because harmonics operate at frequencies higher than that associated with the fundamental frequency, harmonic currents increase the voltage drop in a circuit compared with the value calculated when using the tables of voltage drop in Appendix 4 of BS 7671, which typically apply to frequencies ranging from 49 to 61 Hz.
However, the increase in voltage drop is more linked with higher harmonic frequencies and for larger conductor sizes, and as an effect from an increase in inductive reactance, which is outside the scope of this article.
Summary
This article has highlighted the issue surrounding triplen harmonics and the considerations for the contractor when installing non-linear loads, typically within larger industrial/commercial type installations. Also considered was the impact of triplen harmonics, the subsequent effects of their combined currents circulating within a neutral conductor of a three-phase, four-wire distribution system, and the effects that may significantly reduce the neutral conductors current-carrying capacity while increasing the risk of overload.
A subsequent article in Connections will consider the sizing of multicore cables and look in more detail at the guidance given in Section 5.5 of Appendix 4 of BS 7671.



